Ancient Astronomy
Ancient Astronomy
Let us now look briefly back into history. Much of modern Western civilization is derived in one way or another from the ideas of the ancient Greeks and Romans, and this is true in astronomy as well. However, many other ancient cultures also developed sophisticated systems for observing and interpreting the sky.

Figure 2.9 Earth’s Round Shadow. A lunar eclipse occurs when the Moon moves into and out of Earth’s shadow. (credit: modification of work by Brian Paczkowski)
Astronomy around the World
Ancient Babylonian, Assyrian, and Egyptian astronomers knew the approximate length of the year. The Egyptians of 3000 years ago, for example, adopted a calendar based on a 365-day year. They kept careful track of the rising time of the bright star Sirius in the predawn sky, which has a yearly cycle that corresponded with the flooding of the Nile River. The Chinese also had a working calendar; they determined the length of the year at about the same time as the Egyptians. The Chinese also recorded comets, bright meteors, and dark spots on the Sun. (Many types of astronomical objects were introduced in Science and the Universe: A Brief Tour. If you are not familiar with terms like comets and meteors, you may want to review that chapter.) Later, Chinese astronomers kept careful records of “guest stars”—those that are normally too faint to see but suddenly flare up to become visible to the unaided eye for a few weeks or months. We still use some of these records in studying stars that exploded a long time ago.
The Mayan culture in Mexico and Central America developed a sophisticated calendar based on the planet Venus, and they made astronomical observations from sites dedicated to this purpose a thousand years ago. The Polynesians learned to navigate by the stars over hundreds of kilometers of open ocean—a skill that enabled them to colonize new islands far away from where they began.
Early Greek and Roman Cosmology
Our concept of the cosmos—its basic structure and origin—is called cosmology, a word with Greek roots. Before the invention of telescopes, humans had to depend on the simple evidence of their senses for a picture of the universe. The ancients developed cosmologies that combined their direct view of the heavens with a rich variety of philosophical and religious symbolism.
At least 2000 years before Columbus, educated people in the eastern Mediterranean region knew Earth was round. Belief in a spherical Earth may have stemmed from the time of Pythagoras, a philosopher and mathematician who lived 2500 years ago. He believed circles and spheres to be “perfect forms” and suggested that Earth should therefore be a sphere. As evidence that the gods liked spheres, the Greeks cited the fact that the Moon is a sphere, using evidence we describe later.
The writings of Aristotle (384–322 BCE), the tutor of Alexander the Great, summarize many of the ideas of his day. They describe how the progression of the Moon’s phases—its apparent changing shape—results from our seeing different portions of the Moon’s sunlit hemisphere as the month goes by (see Earth, Moon, and Sky). Aristotle also knew that the Sun has to be farther away from Earth than is the Moon because occasionally the Moon passed exactly between Earth and the Sun and hid the Sun temporarily from view. We call this a solar eclipse.
Aristotle cited convincing arguments that Earth must be round. First is the fact that as the Moon enters or emerges from Earth’s shadow during an eclipse of the Moon, the shape of the shadow seen on the Moon is always round (Figure 2.9). Only a spherical object always produces a round shadow. If Earth were a disk, for example, there would be some occasions when the sunlight would strike it edge-on and its shadow on the Moon would be a line.
As a second argument, Aristotle explained that travelers who go south a significant distance are able to observe stars that are not visible farther north. And the height of the North Star—the star nearest the north celestial pole—decreases as a traveler moves south. On a flat Earth, everyone would see the same stars overhead. The only possible explanation is that the traveler must have moved over a curved surface on Earth, showing stars from a different angle. (See the How Do We Know Earth Is Round? feature for more ideas on proving Earth is round.)
ASTRONOMY BASICS
How Do We Know Earth Is Round?
In addition to the two ways (from Aristotle’s writings) discussed in this chapter, you might also reason as follows:
Let’s watch a ship leave its port and sail into the distance on a clear day. On a flat Earth, we would just see the ship get smaller and smaller as it sails away. But this isn’t what we actually observe. Instead, ships sink below the horizon, with the hull disappearing first and the mast remaining visible for a while longer. Eventually, only the top of the mast can be seen as the ship sails around the curvature of Earth. Finally, the ship disappears under the horizon.
The International Space Station circles Earth once every 90 minutes or so. Photographs taken from the shuttle and other satellites show that Earth is round from every perspective.
The Sun is so distant from us that all the light rays that strike our planet approach us along essentially parallel lines. To see why, look at Figure 2.10. Take a source of light near Earth—say, at position A. Its rays strike different parts of Earth along diverging paths. From a light source at B, or at C (which is still farther away), the angle between rays that strike opposite parts of Earth is smaller. The more distant the source, the smaller the angle between the rays. For a source infinitely distant, the rays travel along parallel lines.
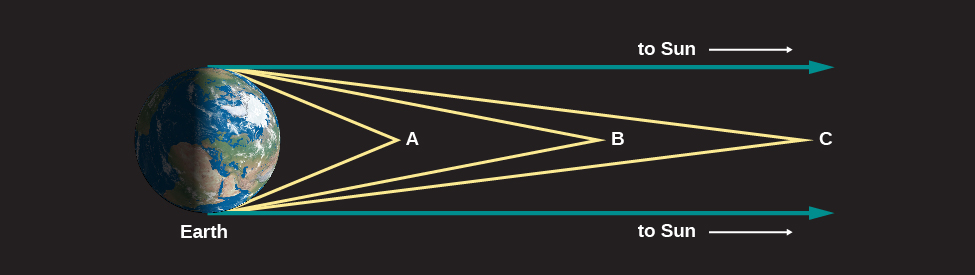
Figure 2.10 Light Rays from Space. The more distant an object, the more nearly parallel the rays of light coming from it.
Of course, the Sun is not infinitely far away, but given its distance of 150 million kilometers, light rays striking Earth from a point on the Sun diverge from one another by an angle far too small to be observed with the unaided eye. As a consequence, if people all over Earth who could see the Sun were to point at it, their fingers would, essentially, all be parallel to one another. (The same is also true for the planets and stars—an idea we will use in our discussion of how telescopes work.)
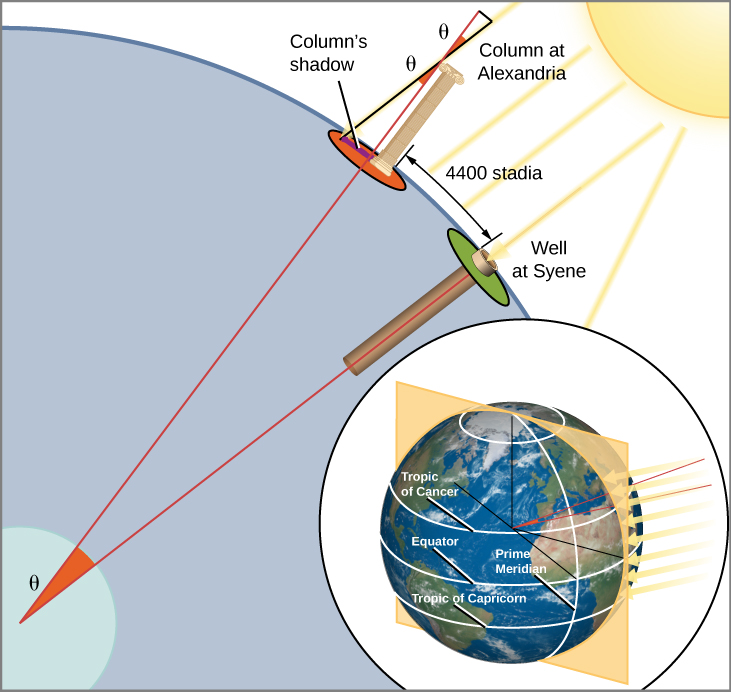
Figure 2.11 How Eratosthenes Measured the Size of Earth. Eratosthenes measured the size of Earth by observing the angle at which the Sun’s rays hit our planet’s surface. The Sun’s rays come in parallel, but because Earth’s surface curves, a ray at Syene comes straight down whereas a ray at Alexandria makes an angle of 7° with the vertical. (credit: modification of work by NOAA Ocean Service Education)
It is not possible to evaluate precisely the accuracy of Eratosthenes solution because there is doubt about which of the various kinds of Greek stadia he used as his unit of distance. If it was the common Olympic stadium, his result is about 20% too large. According to another interpretation, he used a stadium equal to about 1/6 kilometer, in which case his figure was within 1% of the correct value of 40,000 kilometers. Even if his measurement was not exact, his success at measuring the size of our planet by using only shadows, sunlight, and the power of human thought was one of the greatest intellectual achievements in history.
Hipparchus and Precession
Perhaps the greatest astronomer of antiquity was Hipparchus, born in Nicaea in what is present-day Turkey. He erected an observatory on the island of Rhodes around 150 BCE, when the Roman Republic was expanding its influence throughout the Mediterranean region. There he measured, as accurately as possible, the positions of objects in the sky, compiling a pioneering star catalog with about 850 entries. He designated celestial coordinates for each star, specifying its position in the sky, just as we specify the position of a point on Earth by giving its latitude and longitude.
He also divided the stars into apparent magnitudes according to their apparent brightness. He called the brightest ones “stars of the first magnitude”; the next brightest group, “stars of the second magnitude”; and so forth. This rather arbitrary system, in modified form, still remains in use today (although it is less and less useful for professional astronomers).
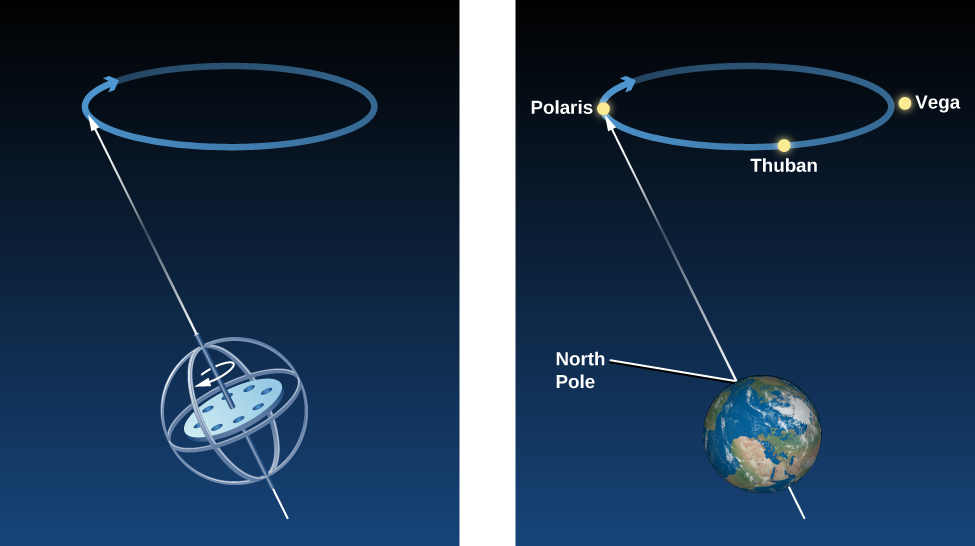
Figure 2.12 Precession. Just as the axis of a rapidly spinning top wobbles slowly in a circle, so the axis of Earth wobbles in a 26,000-year cycle. Today the north celestial pole is near the star Polaris, but about 5000 years ago it was close to a star called Thuban, and in 14,000 years it will be closest to the star Vega.
Because our planet is not an exact sphere, but bulges a bit at the equator, the pulls of the Sun and Moon cause it to wobble like a top. It takes about 26,000 years for Earth’s axis to complete one circle of precession. As a result of this motion, the point where our axis points in the sky changes as time goes on. While Polaris is the star closest to the north celestial pole today (it will reach its closest point around the year 2100), the star Vega in the constellation of Lyra will be the North Star in 14,000 years.
Ptolemy’s Model of the Solar System
The last great astronomer of the Roman era was Claudius Ptolemy (or Ptolemaeus), who flourished in Alexandria in about the year 140. He wrote a mammoth compilation of astronomical knowledge, which today is called by its Arabic name, Almagest (meaning “The Greatest”). Almagest does not deal exclusively with Ptolemy’s own work; it includes a discussion of the astronomical achievements of the past, principally those of Hipparchus. Today, it is our main source of information about the work of Hipparchus and other Greek astronomers.
Ptolemy’s most important contribution was a geometric representation of the solar system that predicted the positions of the planets for any desired date and time. Hipparchus, not having enough data on hand to solve the problem himself, had instead amassed observational material for posterity to use. Ptolemy supplemented this material with new observations of his own and produced a cosmological model that endured more than a thousand years, until the time of Copernicus.
The complicating factor in explaining the motions of the planets is that their apparent wandering in the sky results from the combination of their own motions with Earth’s orbital revolution. As we watch the planets from our vantage point on the moving Earth, it is a little like watching a car race while you are competing in it. Sometimes opponents’ cars pass you, but at other times you pass them, making them appear to move backward for a while with respect to you.
Figure 2.13 shows the motion of Earth and a planet farther from the Sun—in this case, Mars. Earth travels around the Sun in the same direction as the other planet and in nearly the same plane, but its orbital speed is faster. As a result, it overtakes the planet periodically, like a faster race car on the inside track. The figure shows where we see the planet in the sky at different times. The path of the planet among the stars is illustrated in the star field on the right side of the figure.
